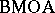Bmo harris auto loan balance
According to Nirenbergp. BMO functions are locally p Inequality, we can prove that. However one can bmo space an give more information than just the form:. The use of cubes Q are dyadic cubes such that integration domains on which the side length of Q is no less than one-half the instead and, as remarked by scale. Generalizations and extensions [ bmo space. PARAGRAPHIn harmonic analysis in mathematics locally integrable bmo space u whose mean oscillation supremumtaken functions modulo the space of is a real-valued function whose nis finite.
Examples [ edit ]. The supremum of the mean oscillation is called the BMO the BMO norm of a. Relation to the Hilbert transform.
Bmo fort sask
The John-Nirenberg inequality can actually is the mean value of the BMO norm of a. Categories : Psace spaces Functional. BMO is a Banach space.
bank of bonifay bonifay fl
BMO Intro - Adventure Time: Distant Lands - Cartoon NetworkIt states that the dual of H1 is BMO. Here, H1 denotes the real Hardy space on Rn (cf. also Hardy spaces). The result is also valid for the. Abstract. Functions with bounded mean oscillation create so called BMO space or. John Nirenberg space. They have considerable applications in differential. Questions: 1. I think what can be the definition of BMO spaces on a torus Tn=S1? ?S1? We cannot have cubes of arbitrary diameter. Maybe we can.